1. Numeracy Preparation Guide For The Vetassess Test
2. Numeracy Preparation Guide For The Vetassess Test Samples

1 Numeracy Preparation Guide for the VETASSESS Test for Certificate IV in Nursing (Enrolled / Division Nursing) course 2 Introduction The Nursing course selection (or entrance) test used by various Registered Training Organisations (RTOs) throughout Victoria, is designed to assess literacy and numeracy at the levels required to successfully complete the Certificate IV in Nursing (Enrolled/ Division Nursing) course. This qualification leads to employment as a Division Registered Nurse in Victoria. The numeracy section is a 0 minute test containing multiple choice questions that assess the ability to work with numbers and to solve problems involving numbers. A calculator is not permitted. In order to assist intending candidates, a revision of the major areas covered in the numeracy test has been included here. It is designed to revise the basic concepts in the light of mental arithmetic methods rather than using a calculator based approach. Each section is based on giving a short explanation of the mathematical theory, worked examples using mental arithmetic methodology, and a small series of practice examples with fully worked answers.

Numeracy Preparation Guide for the VETASSESS Test for Certificate IV in Nursing (Enrolled / Division Nursing) course Introduction The Nursing course.

1. This preparation guide is designed to help candidates who need to take a Reading, Numeracy or Writing skills test for course-entry purposes (specifically diploma entry level).
2. Numeracy vetassess nursing literacy preparation guide numeracy preparation guide for the vetassess test for certificate numeracy preparation guide.

If prospective candidates find any particular area of difficulty it is suggested that they should seek further tutoring, prior to attempting the test. VETASSESS wishes you all the best for your future career endeavours. 3 Contents Topics. Revision of basic concepts: basic arithmetic.

Manipulating fractions I: Finding the smallest denominator. Manipulating fractions II: Increasing the size of the denominator. Manipulating fractions III: Adding and subtracting fractions. Manipulating fractions IV: Improper fractions. Simplifying improper fractions. Creating improper fractions.

Manipulating fractions V: Review of adding and subtracting fractions. Manipulating fractions VI: Multiplying fractions. Fractions to decimals. Decimals to fractions. Percentage A. Decimals to percentage. Fractions to percentage.

Percentage to decimals. Percentage to fractions. Revision of basic concepts A.

Note: The relationship of basic arithmetic and algebra. Believe it or not, many people who find algebra difficult to impossible do so because of a result of a problem with the mathematics described in this first section. If at the end of this revision session of basic arithmetic you still have questions - it is recommended that further tutoring should be sought - a working understanding of 'the order of operations' can work miracles when coming back to algebra! Without a calculator, do the following sum: x? How many of you came up with the answer 0?

How many of you came up with? Is the 'right' answer. In mathematics there is a fundamental rule that for any one calculation, there can only be one answer. Therefore, x MUST have only one answer. The grammar of mathematics (sometimes referred to as the 'order of operations') is to follow the following order in any calculation. Brackets first. Divisions - you do the before dividing.

Subtractions last A simple method of remembering this is the anagram BODMAS. Examples: eg (a) ( ) x ( )? Inside brackets first: ( ) x ( ) x ii. Finish off: x eg (b)? Inside brackets first - do the 'overs' inside the brackets before anything else: 6? Still inside the brackets - do the divisions next:? ( ) 5 iii.

Finish off x eg (c) 0 ( )? Inside brackets first: - do the 'overs' inside the brackets before anything else: 6 ( )? 6 - to finish al the brackets: ( )? - brackets are finished, so it can now be written: x x x? Multiplications next: x x x 0 0? Finish off: 0 (By the way, you should now be able to explain why x ).

Now try these: a. (6 ) - ( x )? Many nursing calculations require the creation and use of fractions.

Many of these calculations involve medication dosages - a mistake here can be fatal! Terminology reminder: The top number in a fraction is called the numerator. The bottom number in a fraction is called the denominator. Manipulating fractions I: Finding the smallest denominator. (Sometimes called 'simplifying' a fraction).

Numeracy Preparation Guide For The Vetassess Test

Consider the following fractions: 7 Each of these fractions represent the same amount, but do you remember how to change from one to the other mathematically? To reduce the size of the denominator without affecting the meaning of the fraction, we divide both the numerator and the denominator by the same number. In the above example: 7, and Another example: 0 00? 0 will divide into both the numerator and the denominator: 0 0?

Now we can see that will divide into the both the numerator and the denominator: 0 6 7 Of course, some of may have noticed a quicker way to find this answer - dividing both the numerator and the denominator by 0 to begin with. Simplifying fractions is always a matter of dividing the same number into both the numerator and the denominator - it's your choice as to what number you start with - so long as it will divide into both. Now try these: a.? Manipulating fractions II: Increasing the size of the denominator. As you will see in the next section, being able to increase the size of the denominator without changing the meaning of the fraction is very useful when adding or subtracting two or more fractions. In the last section, we 'simplified' a fraction by dividing both the numerator and the denominator by the same number.

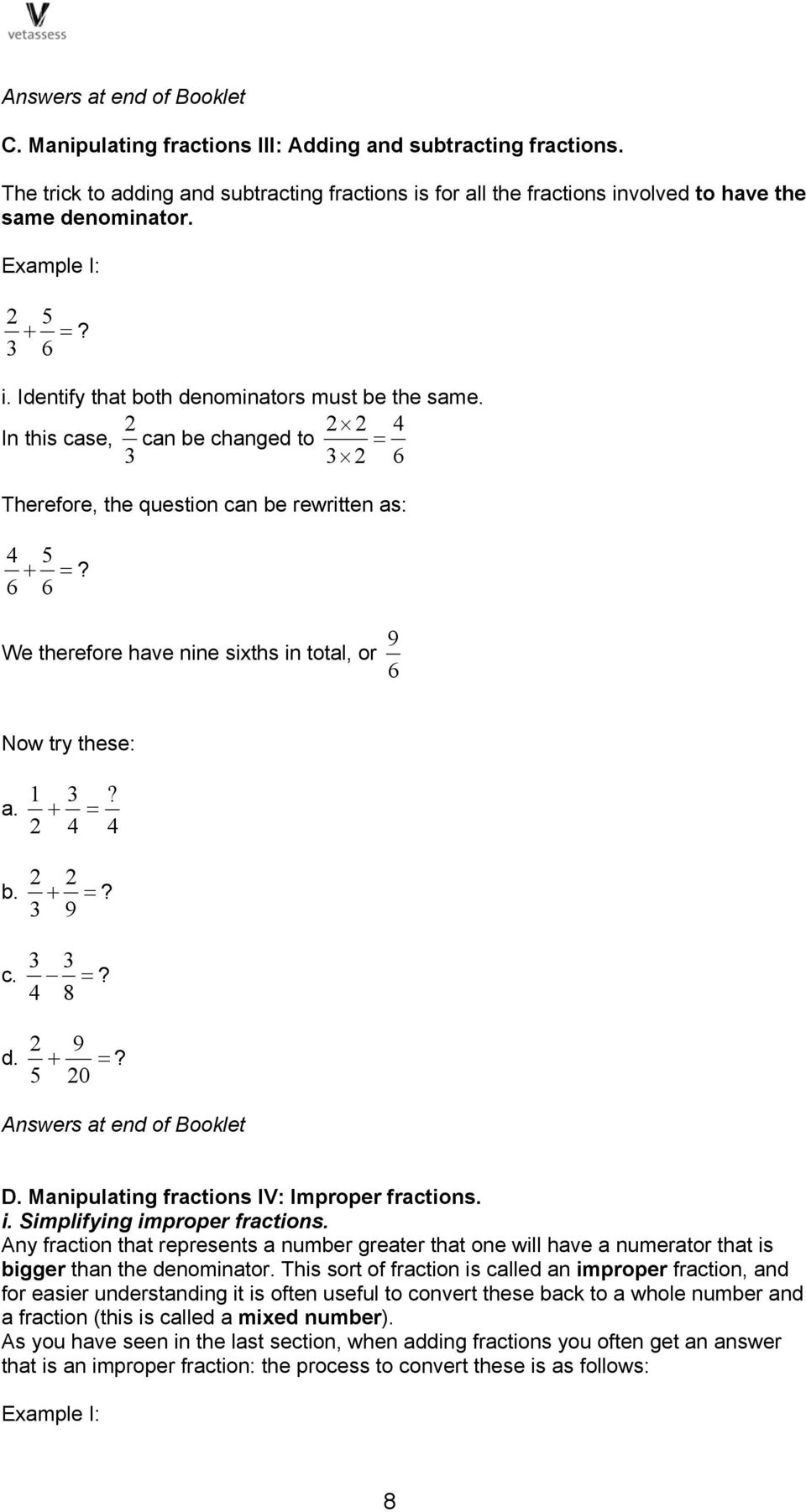
If we want to increase the size of the denominator without affecting what the fraction represents, we simply multiply both the numerator and the denominator by the same number. Because x, we also multiply the numerator by, ie: Now try these: a. Manipulating fractions III: Adding and subtracting fractions. The trick to adding and subtracting fractions is for all the fractions involved to have the same denominator.

Identify that both denominators must be the same. In this case, can be changed to 6 Therefore, the question can be rewritten as:?

6 6 We therefore have nine sixths in total, or 6 Now try these: a.? Manipulating fractions IV: Improper fractions. Simplifying improper fractions.

Any fraction that represents a number greater that one will have a numerator that is bigger than the denominator. This sort of fraction is called an improper fraction, and for easier understanding it is often useful to convert these back to a whole number and a fraction (this is called a mixed number). As you have seen in the last section, when adding fractions you often get an answer that is an improper fraction: the process to convert these is as follows: Example I: 8 9? Note: Any fraction with the same numerator and denominator always equals. Therefore we can write the above example as follows:? Now try these: a.? Manipulating fractions IV: Improper fractions.

(continued) ii. Creating improper fractions from mixed numbers. A mixed number is where you have a whole number and a fraction together, eg There are also times when performing calculations that you need to turn a mixed number into an improper fraction.

Remembering that a fraction with the same numerator and denominator always equals one. Therefore: This can now be added just like any other addition of fractions question to give: 0 Now try these: a. Manipulating fractions V: review of adding and subtracting fractions. Having completed the previous four sections, now try the following problems as a review: a. Multiplying fractions. From time to time, a calculation will call for multiplying two fractions together.

To use a simple real world example - you have half a pizza wish to eat half of this now and save the rest for later. How much pizza will you eat now? Intuitively, you know this is one quarter of the pizza. Mathematically this is: Example I: Perhaps you have quarter of a pizza, and wish to eat half of it - again intuitively this is one eighth.

Mathematically this is: Example II: 8 The simple rule to remember when multiplying fractions is to multiply the numerators together, and the denominators together: For Example I: and for Example II: 8 Example III: You have two thirds of a pizza, and wish to divide it into two. How big is each piece? Simplifying: Now try these: (Simplify the answers where you can) a.? Decimals These are a special sort of fraction, where the denominator is limited to multiples of 0. To differentiate them from all other fractions, they are written in a different form: 0.

Is really 0 0. Is really is really 000 A. Fractions to decimals: Converting fractions to decimals involves nothing more than a conversion of a fraction to have a denominator that is a multiple of 0: Example I: Convert to a decimal.

Since will divide into 0, we can do the following: 0 in decimal notation this is 0. Example II: 0.?

Will not divide into 0, but it does divide into 00, therefore: 0. Example III:? 00 does not divide neatly into 0, 00 or even so what can you do? 13 In this case, you need to remember a set of basic fraction to decimal conversions.

The smallest number of these you can get away with are listed below: (NB: These MUST be committed to memory). Fraction Decimal Shortcuts. To get the decimal equivalent of, multiply by ( x ). For, multiply by ( x ). If you remember all of these, you may never need to do the calculations given in the examples under 'converting fractions to decimals'. Now try these: a.?

Numeracy Preparation Guide For The Vetassess Test Samples

Decimals to fractions: Since a decimal is nothing more than a fraction using a denominator that is limited to a multiple of 0 (ie. 0, 00, 000 etc), it is easy to convert them: Example I: Express 0. As a fraction. Out of 0 parts, or 0 Example II: Express 0.6 as a fraction.

Step i parts out of 000, or 000 Step ii. This can be simplified as will divide into the numerator and denominator: Step iii.

This too can be simplified by dividing the numerator and denominator by: Step iv. This too can be simplified by dividing the numerator and denominator by: 7 0 This is the simplest form for this fraction. Now try these: Express the following decimals as fractions: a. Percentage This too is just a special sort of fraction, but this time the denominator is always 00. To differentiate a percentage from a decimal or a fraction, percentages are written as 'how many parts out of 00' followed by the symbol%. Percent (%) is really the fraction percent (0%) is really the fraction percent (00%) is really the fraction 00 A. Decimals to percentage: Multiply the decimal by 00 Example I: Express 0.6 as a percentage 0.6 x 00 6% Example II: Express 0.

As a percentage 0. X 00.% Now try these: Express the following decimal numbers as percentages: a. Fractions to percentage: Step: convert the fraction to a decimal value (as described in section, part A). Step: multiply the decimal value by 00. Example: Express as a percentage as a percentage: x% 16 Now try these: Express the following fractions as percentages: a.

Percentage to decimals: Step: Divide the percentage by 00. Step: Express in decimal notation. Example: Express% as a fraction.

Step: Divide the percentage by 00% 00 Expressed in decimal notation: 0. Example II: Express.8% as a decimal. Step: Divide the percentage by% 00 This however is a mixed fraction and decimal. We need to turn it into complete fractional notation. To do this, we convert.8 to a whole number. If we multiply.8 x 0 we get 8, and using our skills in fractions covered earlier, we know that we need to also do the same to the denominator: Step: Express in decimal notation.8% 0.8 6 17 Now try these: Express the following percentages as decimals: a.

0% b.% c.% d.% D. Percentage to fractions: This is the reverse process of section B above: Step: Divide the percentage by 00. Step: Convert the decimal to a fraction. (as described in section, part B). Step: simplify the fraction (if possible). Example I: Express 0% as a fraction: Step: Divide the percentage by Step: Convert the decimal to a fraction: Step: simplify Now try these: Express the following percentages as fractions: a.% b.% c.

6.7% 7 18 Answers for Sections to. Revision of basic concepts: basic arithmetic.

(6 ) - ( x ) Fractions A. Manipulating fractions I: Finding the smallest denominator. Manipulating fractions II: Increasing the size of the denominator. Manipulating fractions III: Adding and subtracting fractions. Manipulating fractions IV: Improper fractions. Simplifying improper fractions.

0 Creating improper fractions. Manipulating fractions V: Review of adding and subtracting fractions. Manipulating fractions VI: Multiplying fractions.

D Decimals A. Fractions to decimals. Decimals to fractions.

Percentage A. Decimals to percentage. Fractions to percentage. A% b% 7 8 c% 0 6 d% 0 00 C. Percentage to decimals.

0% b.% 0.0 c.% 0. Percentage to fractions. 6% 0 d.% 000 e.

This kit is for students wishing to become agents' representatives (in Victoria only), and incorporates the following units of competency:. (CPPDSM4007A) Identify legal and ethical requirements of property management to complete agency work.

(CPPDSM4008A) Identify legal and ethical requirements of property sales to complete agency work. (CPPDSM4080A) Work in the real estate industry. The kit also includes a Forms Pack. Legislation extracts relevant to practice in Victoria and two Consumer Affairs Victoria information booklets for property management and sales.